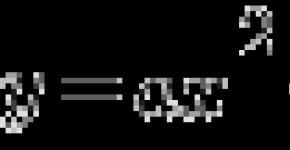Additive and multiplicative measurement errors. Additive and multiplicative error

According to the above absolute error from the values \u200b\u200bof the measured value, errors are distinguished:

● Additive Δ A, independent values;

● multiplicative Δ m, which are directly proportional to the measured value;

● Nonlinear Δ H, having a nonlinear dependence on the measured value.

These errors are used mainly to describe the metrological characteristics of SI. The separation of errors to additive, multiplicative and non-linear is very essential when solving the issue of rationing and the mathematical description of the errors of the SI.

Examples of additive errors - from constant cargo on a cup of scales, from inaccurate installation to zero appliance arrows before measuring, from thermo-emfs in DC circuits. The causes of the emergence of multiplicative errors can be: change the gain of the amplifier, changing the rigidity of the meter gauge membrane or the springs of the device, changing the reference voltage in the digital voltmeter.

These varieties of errors are sometimes called as follows:

● Additive ---- zero error;

● multiplicative ----- The error of the steepness characteristics;

● Nonlinear --------- The error of nonlinearity.

Due to the fact that the additive and multiplicative components are characteristic of the measurement tool, and in the range of measured values, then based on the specified true (valid) linear size of the design element (14.3 cm), assume that the used measurement means allows The measurements in the range from 0.1 cm to 25 cm, and the one for the entire scale of the average relative error is 12.7%, which is calculated by formula (2.5) in the 2nd section of this work. Based on the selected measurement range of the measurement means (0.1cm - 25 cm), we take from it, for example, 10 equivalent fixed (reference) values \u200b\u200bof the linear size of the design element, including the specified true (valid) value equal to 14.3 meters. As a result, a number of measured reference values \u200b\u200bof linear dimensions L. at I.used by means of measurement will be viewed: 2.5; five; 7.5; 10; 12.5; fifteen; 17.5; twenty; 22.5; 25 (cm).

Using expression (2.5), you can determine the values \u200b\u200bof the total absolute error for all members of the row ( L. at I.), namely:

(3.1)

Calculated values \u200b\u200bof the total absolute error Δ with I. For all members of the series, taking into account the rules for rounding the measurement results and measurement errors (given in Appendix 1), are presented in Table 3.1.

Table 3.1.

The results of calculations of the total, additive and multiplicative

absolute errors

No. member number	L. at I,m.	, %	Δ S. I., cm	Δ A, cm	Δ m, see
	2,5	12,7	0,318	0,318
		12,7	0,635	0,318	0,318
	7,5	12,7	0,953	0,318	0,635
		12,7	1,270	0,318	0,952
	12,5	12,7	1,588	0,318	1,27
		12,7	1,905	0,318	1,587
	17,5	12,7	2,223	0,318	1,905
		12,7	2,540	0,318	2,222
	22,5	12,7	2,858	0,318	2,54
		12,7	3,175	0,318	2,857

Using the results of calculations of the total absolute error Δ with I. and a number of measured reference linear dimensions L. at I., a graph is built (see Fig. 3.2) dependencies At the same time, the points for which it is built is constructed. On the graphs of the graph, the initial and final values \u200b\u200bof the measurement range of the measurement means (LAN \u003d 2.5 cm and the LEX \u003d 25 cm) and the maximum value of the total error Δ С (δ Ск \u003d 3,175 cm).

Fig. 3.2. Schedule of the total absolute error

On the resulting graph (Fig. 3.2), an additive component (Δ A) of the total absolute error (Δ C) is distinguished, which is equal to the total absolute error with a minimum (initial) value of the reference values \u200b\u200bof linear dimensions (at the beginning of the SI measurement range), i.e. Δ A \u003d 0.318 cm.

A graph is built (Fig. 3.3) dependences of the absolute additive error Δ A \u003d f.(L. Fl. I.), which is a straight parallel axis of the abscissa passing from a point with the ordinate Δ A \u003d 0.318 cm.

Fig. 3.3. Graph absolute additive error

On the resulting graph (see Fig. 3.2) dependences Δ I.= f.(L. ET), a graph of the multiplicative component Δ m \u003d is distinguished f.(L. At). The results of the calculation of the absolute multiplicative error are shown in Table 3.1, and the chart in Figure 3.4.

Fig. 3.4. The graph of the absolute multiplicative error

Based on the fact that the used measurement means has one for the entire scale of the average relative error Δ CP 12.7%, which is calculated by formula (2.5) in the 2nd section of this work and was used to highlight the additive and multiplicative components of measurement errors in this section Work, then the graph of this error will be a horizontal straight line with a residency of 12.7% for the entire range of changes in linear size L ET.

Calculate the relative additive components of the error (Δ A I.) For each measurement measuring means, using the obtained value Δ A \u003d 0.318 cm and the dependence of the form:

Results of calculations of relative additive components of errors (Δ A I.) Presents in Table 3.2, and the chart in Fig.3.5.

Using the results of calculations of the absolute multiplicative component of the error, which are shown in Table 3.1, calculate the relative additive components of the error (Δ m I.) For each measurement measurement, using the dependence of the form:

Results of calculations of relative multiplicative components of errors (Δ m I.) are presented in Table 3.2, and the graph in fig. 3.6.

Table 3.2.

Results of calculations of relative components of measurement errors

No. member number	L. at I,cm	Δ Wed, see	Δ A. I,cm	Δ M. I,cm
	2,5	12,7	12,72	0,0
		12,7	6,36	6,3
	7,5	12,7	4,24	8,5
		12,7	3,18	9,5
	12,5	12,7	2,544	10,2
		12,7	2,12	10,6
	17,5	12,7	1,8	10,9
		12,7	1,6	11,1
	22,5	12,7	1,4	11,3
		12,7	1,3	11,4

Fig. 3.5. Schedule relative additive error

Fig. 3.6. Schedule relative multiplicative error

CONCLUSIONS

Performed testing allowed:

1) make the calculation of the absolute, relative and reduced errors of the results of measurements of the linear size of the construction of the building under construction, the mean values \u200b\u200bof which were respectively:

Δ cp \u003d 1.82 cm, %, .

2) calculate and build graphs of the total absolute and relative errors of the results of measurements of the linear size of the structure of chemical equipment, to highlight from them and build graphs of additive and multiplicative components of errors;

Any measurement tool possesses a static characteristic, i.e. A characteristic that functionally connects the output value y with an input value of X. Typically, the static characteristic is linear. In the absence of errors, the ratio is true for it.

where Y. n - nominal static characteristics of measuring instrument; S. N - Nominal sensitivity Measurement.

The presence of error of the measurement means causes a change in sensitivity ( S. H + D. S.), as well as the displacement of the measurement result by the value of D a, i.e.

Y.= (S. H + D. S.) × X.+ D a.

Error D. Y. The measurement results are determined as

D. Y.= Y.– Y. H \u003d D. S.× X +.D a.

The first component of the error is multiplicative (D M \u003d D S.× X.), and the second is additive (D a \u003d d a).

We give the definition of additive and multiplicative errors.

Additive called error absolute value which is invariably in the entire range of the measured value.

A systematic additive error displays the nominal characteristic parallel to up or down by ± d A A (Fig. 5.2).

An example of a systematic additive error is the error from the inaccurate installation of the device to zero, from the contact e.D. in DC circuits. An additive error is also called zero error.

Multiplicative Call error absolute value which changes in proportion to the measured value.

With a systematic multi-plicent error, the actual characteristic deviates from but-mining up or down (Fig. 5.3).

Examples of systematic multiplicative errors are errors due to changes in the fission coefficient of the voltage divider, due to the change in the rigidity of the spring of the measuring mechanism, etc. A multiplicative error is also called the sensitivity error.

In the measurement tools, additive and multiplicative errors are usually present at the same time. In this case, the resulting error is determined by the sum of the additive and multiplicative errors D \u003d D A + D M \u003d D A + D M × H.where D M is a relative multiplicative error. Depending on the ratios of the additive (D A) and the multiplicative (D M) errors of the accuracy of the measurement tools are designated differently. It is possible to distinguish three characteristic cases of the ratio of these errors 1) d a \u003d 0, d m ¹ 0; 2) d a ¹ 0, d m \u003d 0; 3) D A @ D m.

1. According to the method of expression of the error:

On absolute;

Relative;

Led.

Absolute errordetermine as the difference between the measured and valid values \u200b\u200bof the measured value (Formula 4):

The absolute error is expressed in units of the measured value.

The absolute error may not serve as an accuracy of the accuracy, since it is independent of the measured value. For example, measurement error \u003d 0.5 mm when measuring length \u003d 100 mm corresponds to a sufficiently high measurement accuracy, and at \u003d 1 mm - low.

Relative error It seems as the ratio of absolute error to the valid value of the measured value. The relative error is found from the relationship (5):

(5)

The relative error is more accurate characteristic and most informative, as it makes it possible to compare the results and evaluate the quality of measurements performed at different times, various means or operators.

However, the relative measurement error cannot be used to normalize the error of measuring instruments, since when the measured value of the measured value is to zero, its minor changes lead to enormous changes.

To eliminate the specified disadvantage, the concept of the above error is introduced.

Limited error- This is the ratio of the value of the absolute error to the constant rational value (Formula 6):

(6)

For normalizing value, either the upper limit of one-sided scale of measurements Either measurement range

2. By character dependence on the measured value Errors are divided into additive and multiplicative.

Additive error(zero error) is the error of measuring instruments remaining constant throughout the measurement range, i.e. The additive error does not depend on the value of the measured value.

Adducive, for example, is the error caused by an inaccurate zero setting from a shooting device with a uniform scale.

Multiplicative error (sensitivity error) is called error of measuring instruments, increasing or decreasing with an increase in the measured value, i.e. The multiplicative error varies in proportion to the measured value.

Animately multiplicative, for example, is the error of measuring time segments by lagging or hurrying hours. This error will increase in absolute value until the watch owner will set them correctly by the exact time signals.

3. By the nature of manifestation Errors are divided into systematic, random and coarse (misses).

In general, the error of the measurement result includes systematic and random components (Formula 7):

where - the systematic component of the overall error - the random component of the overall error (the coarse error is part of the random component).

Systematic error Measurements are called the component of the measurement result of the measurement, which, during repeated measurements of the same value in the same conditions, remains constant or naturally changes, usually progressing.

Systematic errors can be called not enough to accurately execute the adopted principle and methods of measurements, constructive disadvantages of measuring instruments.

To systematic constant The errors (remaining constant during repeated measurements) include the error caused by the temperature deformation of the measured part, and the error of the measurement means when the temperature is deviated from normal conditions.

Example systematic progressive Error (naturally changing during repeated measurements) is the error caused by the wear of the measuring tip of the measurement tool during contact measurements.

A distinctive feature of systematic errors is the predictability of their behavior. Since they distort the measurement result, they need to be eliminated by the introduction of amendments or adjust the instrument with bringing systematic errors to a permissible minimum.

Amendment -this value of the value entered into the unfailed measurement result in order to eliminate the components of the systematic error. By the introduction of the amendment, as a rule, a systematic constant error of measuring instruments.

With the introduction of amendment, the measurement equation will be viewed (Formula 8):

where - indication of measuring instruments; - the value of the measured value; - systematic measurement error; - amendment.

The amendment is numerically equal to the value of the systematic error and is opposite to it by sign .

The value obtained by measuring the value of the magnitude and refined by the introduction of the necessary corrections to the action of systematic errors are called fixed measurement result.

Systematic errors in the case when they are known and the values \u200b\u200bof them in the form of amendments are indicated in the regulatory and technical documentation (passport) on the measurement means, should be taken into account in each of the measurement results.

Systematic constant errors can also be detected (detected) by comparing measurement results with other, obtained more accurate methods and means.

In some cases, it is possible to get rid of systematic errors in whole or in part during the measurement process even when they are unknown or by magnitude or by the sign. For example, when compensated for a sign, the measurement is organized in such a way that the systematic error entered once with one sign, and another time - with the opposite. Next, they take the arithmetic average of two results - while the systematic error is excluded.

Random errormeasurements are called the component of the error of the measurement result, which, with repeated measurements of the same value in the same conditions, changes in an unforeseen, randomly.

The reasons causing random errors, many, for example, the battery of the elements of the device, fluctuations in the ambient temperature, rounding the instrument readings, the change in the attention of the operator, etc.

In the manifestation of these errors, there are no patterns, they are detected during repeated measurements of the same value as a certain scatter of the resulting results.

Random errors are inevitable, unrelated and always present as a result of the measurement. Unlike systematic random errors, it is impossible to exclude from the measurement result by the introduction of amendments, but they can be significantly reduced by increasing the number of single dimensions. This makes it possible using the methods of probability theory and mathematical statistics, clarify the result, i.e. Apply the value of the measured value to the true one.

A random error of the measurement result also includes slip or rough error.

Promach (coarse error) The error is called the measurement result included in a number of measurements, which for these conditions is sharply different from the other results of this series.

Damage, as a rule, arise due to errors or incorrect operation of the operator, the incorrect reference of the instrument readings, sharp short-term changes of the conditions during measurements, etc. The moment of the occurrence of misses for the experimenter is case and unknown. In repeated measurements, the set of results obtained may contain several results having rude errors in its composition.

If blunders are detected during the measurement process, the results containing them are discarded as unreliable. As a rule, the identification of misses is made on the basis of the analysis of measurement results with the help of various probabilistic criteria.

The separation of errors to systematic and random is of great importance in the development of methods for reducing errors, but not always easily feasible. Sometimes, depending on the method of performing the same measurement, the error of the result can be both systematic and random.

4. By source of appearancethe errors are divided into methodical, subjective and instrumental.

Methodical error (error of the measurement method) -this is a component of measurement errors due to the shortcomings of the theory or measurement method.

This error occurs due to: admitted simplifications during measurements, due to the inaccuracy of transmitting the size of the size from the object to the measurement facility, the error of data processing, etc.

Methodical methods also include components of the error due to the limited accuracy of the formulas used to find the measurement result, and the imperfections of the receptions, with the help of which the principle of measurements are implemented. An example of such an error is an indirect measurement of electrical resistance based on the Ohm law (using an ammeter and voltmeter). Depending on the connection of instruments, the readings of one or another contain systematic errors, which causes the error of the result.

In most cases, methodical errors are systematic, but they are also randomly manifestation. For example, if the measurement method equations include coefficients depending on the measurement conditions that change randomly.

The main feature of the methodological errors is the fact that they cannot be specified in the instrument passport, but should be evaluated by the experimenter itself, i.e. Methodical errors do not depend on the quality of the manufacture of measuring instruments.

Subjective error (reference error, personal error) - This is the component of the measurement error depending on the operator.

This error is due to the individual characteristics of the operator (inattention, disadvantage or absence of qualifications), the effect of the heat emission of the operator on the measurement means.

Such an error is manifested in cases where reading and fixing (registration) of observation results are carried out either by the operator or automatically; Their main reason is inaccuracy, rounding the counts.

Subjective errors can not be indicated in the passport on the measurement means. Therefore, in order to avoid them, it is necessary to comply with the rules of operation of measuring instruments, increase the skills of working with measuring equipment and improve counting devices.

Instrumental error (dashboard, hardware)- This is a component of measurement errors due to the error of the measurement tool used.

This error is determined by the imperfection of measuring instruments, constructive and technological limitations, the influence of external conditions.

The instrumental error includes the error of measurement tools and the error of interaction of measurement means with the object.

The error of the interaction of measuring instruments with the object arises due to the fact that the transmission of information is always associated with the selection of some energy from the object. The interaction of measuring instruments with an object may be different in physical nature: mechanical, electric, thermal, etc. However, in any case, it is associated with the energy exchange between the object and the means of measurements occurring in time and space.

Tool errors are also usually addicted to the input of the measurement tools caused by its connection to the measurement object. For example, when the measuring device is turned on, the mode of operation of this chain changes in the electrical circuit.

It is necessary to distinguish the error of measuring instruments and measurement error. The error of measurement means is only part of the measurement error.

5. Under the conditions of the use of measuring instrumentserrors are divided into basic and extra.

Basic error - error of measuring instruments in normal (laboratory) conditions of application, due to the properties of measuring instruments.

These conditions are established by regulatory documents for the types of measuring instruments or individual types of them. The establishment of conditions of application and especially normal conditions is very important to ensure the uniformity of the metrological characteristics of measuring instruments.

The main error may include error variation , manifested in the difference in the measurement tool readings in the same point of the measurement range at different directions of the approach to this point; error of graduation due to the errors of the exemplary tools used in the process of measurement means; quantization error - rounding operation in digital measuring instruments.

Additional error - component of the error of measuring instruments, arising in addition to the basic error due to the deviation of any of its influencing values \u200b\u200bfrom its normal value or due to its output beyond the normal range of values.

For example, under operating conditions when installing the measuring instrument on an airplane, it will have to work with a change in the ambient temperature in the range of ± 50 ° C, pressure from 10 2 Pa to 10 4 MPa, the supply voltage by 20%, which will cause errors that significantly exceed the main .

The main and additional errors are determined in static mode, so they relate to static errors that will be considered in the next paragraph.

6. Under the terms of the change of the measured value Errors are divided into static and dynamic.

According to the dependence of the absolute error from the values \u200b\u200bof the measured value, the errors are distinguished (Fig. 3.1):

· Additive, independent values;
· Multiplicative, which are directly proportional to the measured value;
· Nonlinear having nonlinear dependence on the measured value.

These varieties of errors are sometimes called as follows:

additive ---- zero error;

multiplicative ----- The error of the steepness characteristics;

nonlinear --------- The error of nonlinearity.

Fig. 3.1.

Due to the fact that the additive and multiplicative components of errors are characteristic of the measurement tool, and in the range of measured values, then based on the specified true (valid) value of the linear size of the design element (17m), assume that the used measurement means allows measurements to The range from 1 m to 100 m, and the one for the entire scale of the average relative error, which is calculated by formula (2.5) in the 2nd section of this work. Based on the selected measurement range of measurements (1m - 100m), take from it, for example, 10 equivalent fixed (reference) linear size values \u200b\u200bof the structure element, including the specified true (valid) value equal to 17 meters. As a result, a number of measured reference values \u200b\u200bof linear dimensions used by measuring means will be: 7; 17; 27; 37; 47; 57; 67; 77; 87; 97 (m).

Using expression (2.5), you can determine the values \u200b\u200bof the total absolute error for all members of the series, namely:

The calculated values \u200b\u200bof the total absolute error for all members of the series, taking into account the execution of rules for rounding the measurement results and measurement errors (given in Appendix 1), are presented in Table 3.1.

Table 3.1.

Results of calculations of the total, additive and multiplicative absolute errors

No. member number

Using the results of calculations of the total absolute error and a number of measured reference values \u200b\u200bof linear dimensions, a graph is built (see Fig.3.2) dependencies, and the points on which it is built. On the axes of the graph, the initial and final values \u200b\u200bof the measurement range of the measurement means (LAN \u003d 1 M and LEX \u003d 100 m) and the maximum value of the total error of D C (d SK \u003d - 11.5 m).

On the resulting schedule (Fig. 3.2), an additive component (D A) of the total absolute error (D C) is distinguished, which is equal to the total absolute error with a minimum (initial) value of the reference values \u200b\u200bof linear dimensions (at the beginning of the measurement range of SI), i.e. D A \u003d - 0.89 m.

A graph (Fig.3.3) of the dependence of the absolute additive error d A \u003d f (L et.i), which is the direct parallel axis of the abscissa passing out of the point with the ordinate d and \u003d -0.89 m.

Fig.3.2.

Fig.3.3.

On the resulting schedule (see cris.3.2) dependences, a graph of the multiplicative component d M \u003d F (L ET), which is parallel to the graphics of the total absolute error, but begins not from the point with coordinates (7; 0.89), and from points with coordinates (7; 0), because , on the string axes, the initial and final values \u200b\u200bof the range of linear dimensional l fl (LAN \u003d 7 m and LEX \u003d 97 m) and the maximum value of the multiplicative error d m (d Mk \u003d 11.5 m). The results of the calculation of the absolute multiplicative error are shown in Table 3.1, and the chart in Figure 3.4.

Based on the fact that the used measurement means has one for the entire scale of the average relative error of -12.7%, which is calculated by formula (2.5) in the 2nd section of this work and was used to highlight the additive and multiplicative components of measurement errors in this section of the work The graph of this error will be a horizontal straight line with the ordinate -10.0% for the entire range of changes in linear size L ET.

Calculate relative additive components of errors () for each measurement measuring using the resulting value.

D A \u003d0.89 M and dependence of the form:

The results of the calculations of the relative additive components of the errors () are presented in Table 3.2, and the chart in Fig.3.5.

Fig.3.4.

Table 3.2.

The results of the calculations of the relative components of measurement errors.

No. member number

Fig.3.5.

Using the results of calculations of the absolute multiplicative component of the error, which are shown in Table 3.1, calculate the relative additive components of the error () for each measurement to the measurement means using the dependence of the form:

The results of the calculations of the relative multiplicative components of the errors () are presented in Table 3.2, and the chart in Fig.3.6.